 If at some point you ever want to buy property near water, a variation of this question will undoubtedly pass through your head: what are the chances that my {insert name of your expensive piece of property close to water} floods?
If at some point you ever want to buy property near water, a variation of this question will undoubtedly pass through your head: what are the chances that my {insert name of your expensive piece of property close to water} floods?
In this post, we will calculate the chance of such an event happening, or rather the chance your beautiful riverside property floods over the life of your 30-year mortgage. We will do this using our understanding of probability and statistics.
Flood events, return periods, and probability
In my experience, the probability of flood exceedance is a great example of where calculating the probability of something not happening is more intuitive and easier to understand. It also shows that there is a fundamental misunderstanding of the vocabulary used to communicate the probability of a flood event. You may have heard something like this before:
"Bob, the heavy rains north of Waterville were responsible for the 100-year flood event downtown."
Or,
"The city updated their flood maps to include our house in the 100-year floodplain."
Do you know what is really meant by the term "100-year flood"? It is NOT the case that this event will only happen once every 100 years.

Of course, these probabilities describe the chance an event happens in a single year. Next, let us calculate the probability of an event occurring over multiple years.
Formulating exceedance probabilities
We must first assume that the probabilities of flooding each year are independent and stationary. Independent means that the probability of occurrence of a certain size flood in one year does not change the probability of a flood in any other year. Stationary means that the probability does not change over time (i.e. in 30 years, the probability and size of a 100-year flood will be the same as today).
Next, we must reconsider the question. We want to know what the probability is of a certain sized flood happening at least once over n consecutive years. The complement of this question would be: what is the probability that a flood of size X does not occur during the next n consecutive years?
In any given year it is 100% likely that it will or will not flood to a certain extent. Therefore, the probability that a flood of size X does not happen is the complement of the probability that it will, or

Because the No-Flood condition, P (X < x), for each year is independent of each other, their joint probability is their product. Thus, the probability that a flood of size X does not occur during n consecutive years is
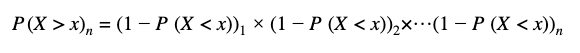
Or,
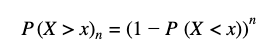
We now have a formula to calculate the probability that a flood of size X will not occur over n consecutive years. Now we can take its complement to determine the probability that a flood of size X will occur at least once over n consecutive years.
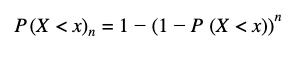
Calculating Exceedance Probabilities
Equation in hand, we can plug in any values we want! The table below shows the probability that a flood of various sizes will occur at least once over a 30-year period. It is essentially guaranteed that you will experience 1-, 2-, and 5-year events. Over 30 years, there is about a 25% chance that you experience a 100-year event.
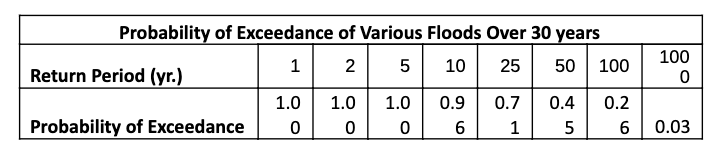
The graph below shows how these probabilities grow over the course of 30 years.
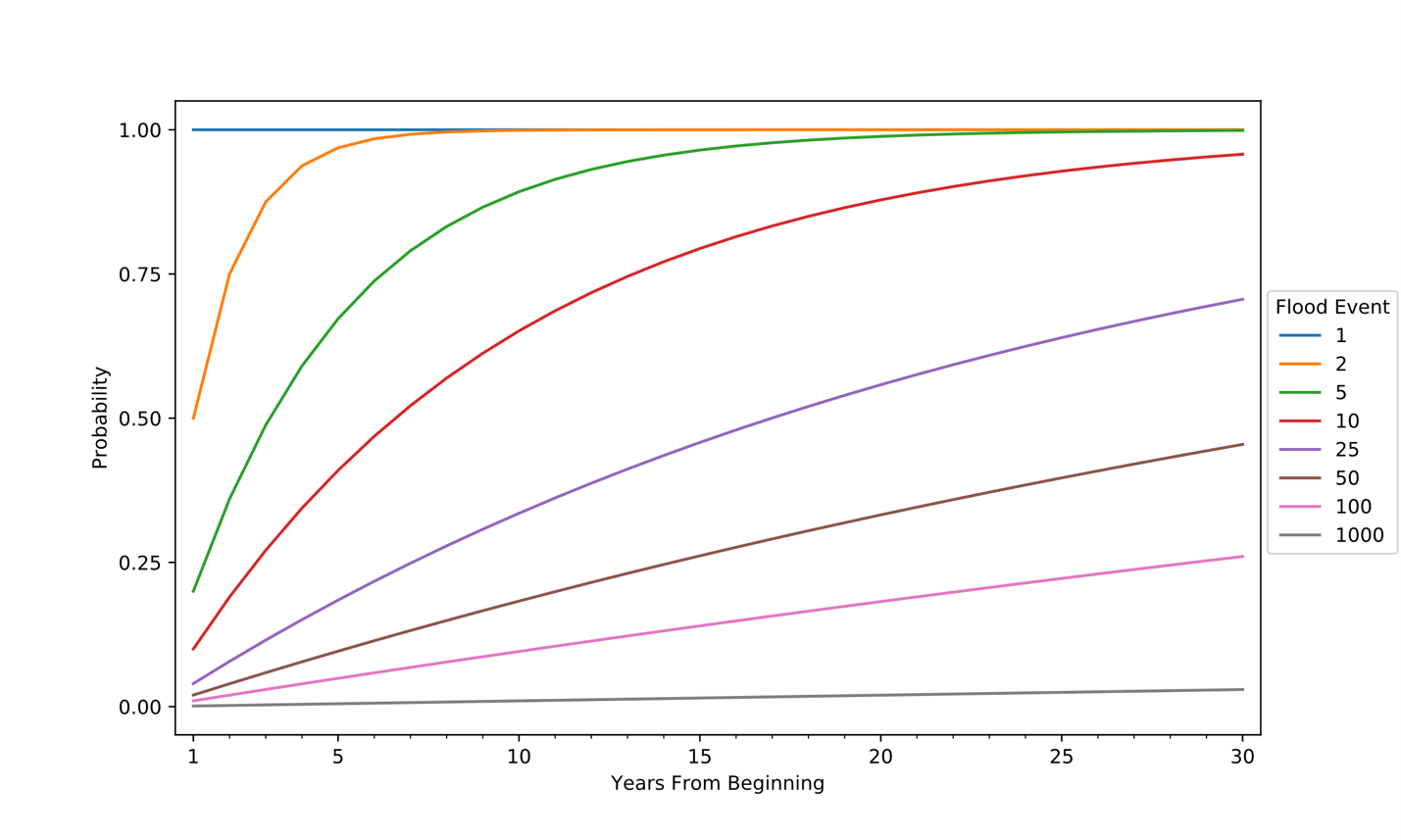
You now can determine the chance that your house will flood over any length of time for any size flood. This, however, will not help you build a castle on a swamp. For that, you will need to consult this guy.
Footnote(s):
You may be asking: how does one estimate the flood extent for an event with a 1% chance of happening every year? Great question! You take historical streamflow data and fit it to a known statistical distribution. You can then estimate the flood extent from the derived distribution.
Extra Credit:
Here’s python code used to make these calculations and the figure above.

Comments