-Jan-19-2021-05-50-28-17-PM.png?width=1080&name=Title_%20How%20to%20Study%20Efficiently%20for%20Hours%20On%20End%20(With%20the%20Help%20of%20a%20Tomato)-Jan-19-2021-05-50-28-17-PM.png) Today, we are going to learn how to solve linear algebraic equations like 3y + 3 = 18 or 5x - 4 = 16. If these equations make you feel a bit queasy, have no fear! I am going to break the process down into five simple steps.
Today, we are going to learn how to solve linear algebraic equations like 3y + 3 = 18 or 5x - 4 = 16. If these equations make you feel a bit queasy, have no fear! I am going to break the process down into five simple steps.
Before we begin, we need to talk about balance. A wise person once said that the secret to life is balance. Believe it or not, that is also the secret to solving algebra equations!
Let’s look at the numerical sentence: 3 + 5 = 4 + 4
Think about what the equal sign is telling us: it signifies that the sum of 3 + 5 is the same as 4 + 4. If we solve each side separately, we can see that is true.
3 + 5 = 8
4 + 4 = 8
We can envision each side of the equal sign as the side of a perfectly balanced scale, like so:

What happens, then, if I add 2 to the left side? The expression would no longer make sense because 3 + 5 + 2 does not equal 4 + 4!
2 + 3 + 5 = 10
4 + 4 = 8
10 ≠ 8
This brings us to one of the most fundamental rules of algebra. Whatever operation you do to one side of the equal sign you must do to the other! Think about it using our scale analogy. If I add or subtract something from one side of the scale, I must do the exact same thing to the other side.


We’re ready to get to our five steps!
Step 1: What am I solving for anyway?
All algebraic equations will ask you to solve for a variable. A variable is a letter that stands in for a value. In an algebraic expression, like 3x + 3, a variable can have many different values. We can just substitute in different values for x. For example, when x = 1 the value of the expression would be 3(1) + 3 = 6. When x = 2, the value of the expression would be 3(2) +3 = 8, and so on.
What makes linear algebraic equations exciting is that, unless they contain absolute values, there is only one value of the variable that can make that equation true. So when you see the phrase “solve for x,” it’s really asking, “What number does x have to be to make this equation true?” To use our scale analogy: what does the variable have to be in order to make the scale balanced?
It is important to identify what you are solving for because you need to get that variable all alone. Your final answer will look something like x = some number.
HOT TIP: Remember that a variable can be ANY letter, it doesn’t have to be x.
For example, if you encounter a problem that says “Solve for y: 3y + 3 = 18,” then your final answer should look like “y = __.”
Likewise, if you encounter a problem that says “Solve for x: 5x - 4 = 16,” then your final answer should look like “x = __.”
Step 2: Identify like terms.
If we want to get our variable alone on one side of the equal sign, we need to get all the other numbers that are not attached to a variable, called constants, together on the other side of the equal sign. In order to do that, we have to identify like terms. A like term is a term that has the same variable and the same coefficient. For example, x2 and 2x2 are like terms, and 8 and 45 are like terms.
Remember that the coefficient, or the number in front of the variable, does not matter. Additionally, it does not matter if a term is positive or negative for two terms to be alike. For example, -4 and 64 are still like terms.
Take a look at the numbers below. Can you circle the like terms?
3 4y3 5 ½ x 14x2 18 -5
If you said that 3, 5, 18, and -5 are like terms, then you are spot on.
If we look at our example problems, we can identify our like terms.
3y + 3 = 18
3 and 18 are like terms
5x - 4 = 16
-4 and 16 are like terms.
HOT TIP: Sometimes it helps to circle or color-code like terms so you can easily see what you need to combine.
Step 3: Combine like terms using inverse operations.
Our next step is to move our constants together to one side of the equal sign and leave our terms with the variable on the other side.
How can I move terms around in my equation? The secret is using inverse operations. In mathematics, every operation has an inverse operation. It’s how you “undo” an operation. For example, if I want to “undo” adding 2, I subtract 2. If I want to “undo” dividing a number by 2, I multiply the number by 2.
Let’s get 3y alone in this example: 3y + 3 = 18.
I subtract 3 from the left side because the inverse operation of adding 3 is subtracting 3. Think about it as “undoing” the operation. Since I subtracted 3 from the left side, I have to do THE EXACT SAME THING to the right side.
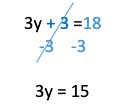
Let’s look at another example: 5x - 4 = 16
The inverse of subtracting four is adding four. So, I add four to both sides.
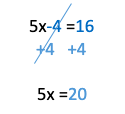
Step 4: Get the variable alone by dividing by the coefficient.
Right now we have 3y = 15 and 5x = 20. What do we still need to do to get the variable all by itself? If you answered “get rid of the coefficient,” then you are exactly right.
It’s important to remember that coefficients are really just a variable multiplied by a constant. For example, another way of writing 5x is 5 times x. Similarly, 3y is the same thing as 3 times y. Therefore, in order to get rid of a number’s coefficient, we just have to perform the inverse operation. What is the inverse operation of multiplication? That’s right, division!
See how it works in our example problems below:
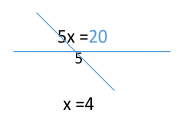
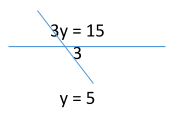
Step 5: Check your answers.
We have our answers! We know exactly what value our variable must be for each equation to make sense. But great mathematicians always check their work.
Checking linear equations is pretty simple: we just have to substitute our answer in for the variable and see if the equation makes sense (AKA see if the sides of the scale are balanced).
Let’s look at our first problem: we found that for the equation 5x - 4 = 16, x must equal 4. We substitute in 4 for x and solve each side according to PEMDAS.
5(4) - 4 =16
20 - 4 = 16
16 = 16
Since each side of the equation has the same value, we know that we are correct!
Try checking the second equation on your own: we found that for the equation 3y + 3 = 18, y = 5.
3(5) + 3 = 18
15 + 3 = 18
18 = 18
Nailed it again! We now can confidently hand in that homework or test, knowing that we have found the correct answer.
Solving linear algebraic equations is challenging at first, but with enough practice, you will master it in no time! Once you feel comfortable with these types of problems, check out my next blog post “Solving Linear Equations with Variables on Both Sides.” See you there!
Mathematics – from elementary school math through high school math – is one of our most frequently requested subjects. Teaching math is notoriously difficult and we maintain a staff of mathematicians who are committed to the art of teaching. There is no course or standardized test that we do not have extensive experience teaching. We work with students who loathe math and students who love it, students who haven’t done math in a decade and students who work on mathematical problems every day.
Looking for middle school support? Check out our other blog posts below!
The Middle School Writers’ Workshop: 3 Steps to a Great Literary Essay Outline
5 Middle School Books for Reluctant Readers
Solving algebraic equations with variables on both sides


Comments